Master Integrals: Formulas & Examples You Need To Know

Noelia Yost 14 May 2025

Do you find yourself wrestling with the enigmatic world of calculus, specifically the art of integration? Then you're in the right place, because mastering integration unlocks a universe of problem-solving possibilities across mathematics, physics, engineering, and beyond.

At its core, integration is the inverse operation of differentiation. While differentiation focuses on finding the rate of change of a function, integration, or anti-differentiation, aims to find the function whose derivative is the given function. Think of it as the process of "undoing" differentiation. The beauty of integration lies in its ability to help us calculate areas, volumes, and countless other quantities that are otherwise inaccessible.

Aspect	Details
Core Concept	The inverse operation of differentiation. It's about finding a function whose derivative is the given function.
Purpose	Used to calculate areas, volumes, and other quantities that reflect accumulated change.
Key Techniques	Integration by Substitution Integration by Parts Trigonometric Integration Partial Fractions
Common Applications	Calculating Areas Under Curves Determining Volumes of Solids of Revolution Modeling Physical Phenomena (e.g., motion, heat transfer) Solving Differential Equations
Reference Website	MathPortal.org (by Milos Petrovic)

The fundamental theorem of calculus provides the crucial link between differentiation and integration. It establishes that integration and differentiation are inverse operations. This means that if you integrate a function's derivative, you'll get back the original function (plus a constant). This theorem forms the foundation upon which the entire edifice of integral calculus is built.

However, directly trying to reverse the differentiation process can be challenging. That's where the techniques and tables of common integrals come in. A solid grasp of these tools makes integration much more manageable and efficient.

Let's delve into some of the most fundamental concepts.

Basic Integrals and Rules

The following are fundamental forms, the building blocks of more complex integral calculations:

The integral of a constant: ′ = −∫ ′ ∫ integral of a constant: ( ) 𝟑 = 𝟑⋅ ( )

Common integrals, indefinite integrals, method of substitution: ∫ f g x g x dx f u du( ( )) ( ) ( )′ = integration by parts.

Here is a more detailed breakdown, alongside practical applications and key insights:

Indefinite Integrals: The Antiderivative

When we calculate an indefinite integral, our result is not a single function but a family of functions that differ only by a constant. That's why we always include "+ c" (the constant of integration) at the end of our answer. Consider the basic example.

The integral of 2x is x + c, where c can be any real number.

Essential Integration Techniques

Now, let's explore some key techniques, with examples:

1. Integration by Substitution

The method of substitution is a powerful technique for simplifying integrals. It works by recognizing that the integral resembles a chain rule application. The core concept is to transform the integral into a simpler form by substituting a part of the expression with a new variable, simplifying the integration process.

Consider an integral of the form: ∫ f (g(x)) g'(x) dx

Let u = g(x). Then, du/dx = g'(x), which implies du = g'(x) dx. Substituting u and du into the integral, we transform it into: ∫ f(u) du

You'll often encounter integrals that involve a function and its derivative. This is a clear indication to try the substitution method. It requires carefully choosing a 'u' and then expressing the original integral in terms of 'u' and 'du'. The goal is to make the integral more manageable. The substitution method is the application of the chain rule in reverse. It helps to simplify integrals where the integrand can be expressed as a composition of functions, combined with the derivative of the inner function.

2. Integration by Parts

Integration by parts is derived from the product rule of differentiation. It's particularly effective when dealing with integrals involving products of functions, like algebraic and exponential functions or trigonometric and algebraic functions. It can be defined as: ∫ u dv = uv - ∫ v du

This method helps in simplifying the product of two functions within an integral. The process involves choosing which part of the integrand will be u and which will be dv. Success depends on selecting u and dv in such a way that the resulting integral is easier to solve than the original.

Trigonometric Identities

Trigonometric identities often play a crucial role in simplifying integrals. These identities can be used to rewrite the integrand in a more convenient form, making the integration process easier. A lot of the time, trig identities assist in transforming an integral to one where substitution is applicable.

Important Trigonometric Integrals

Here are some common integral forms with trigonometric functions:

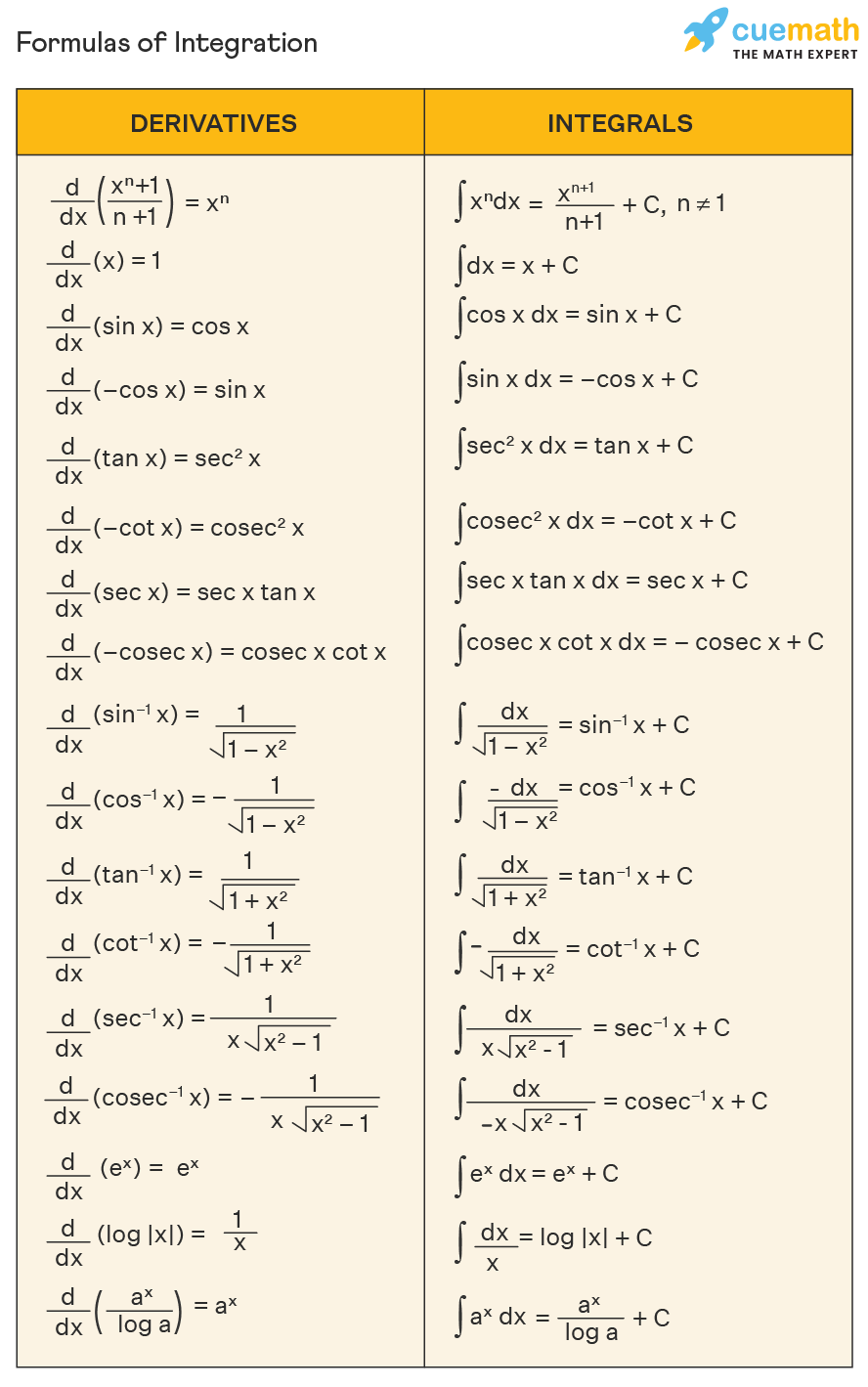
∫ sin(x) dx = -cos(x) + C
∫ cos(x) dx = sin(x) + C
∫ tan(x) dx = ln|sec(x)| + C
∫ sec(x) dx = tan(x) + C

Integrals of Rational Functions (Partial Fractions)

Rational functions are fractions where both the numerator and the denominator are polynomials. Partial fraction decomposition is a technique used to integrate such functions.

The process involves breaking the rational function down into simpler fractions, each of which is easier to integrate. The key to partial fractions is the ability to factor the denominator of the rational function. After that, the original function can be broken down into fractions, which will be simpler to integrate.

Integrals Involving Specific Forms

Certain integral forms appear repeatedly in calculus, and its useful to memorize or have readily available tables for these. These are often linked to trigonometric substitutions and the specific forms.

Integrals involving a + u, a > 0: These integrals often lead to a tangent substitution.Integrals involving u - a, a > 0: These often lead to a secant substitution.Integrals involving a - u, a > 0: These often lead to a sine substitution.Integrals involving 2au - u, a > 0.

Definite Integrals and Areas

Definite integrals are evaluated over a specific interval. They yield a numerical value, representing the net area under a curve between the limits of integration.

To evaluate a definite integral:

Find the antiderivative of the function.
Evaluate the antiderivative at the upper and lower limits.
Subtract the value at the lower limit from the value at the upper limit.

The Fundamental Theorem of Calculus is central to evaluating definite integrals, as it connects the antiderivative to the area.

Applications of Integration

The applications of integration are vast.

Area Calculation: Finding the area under a curve is one of the primary applications.Volumes of Revolution: Integration can determine the volume of a solid generated by revolving a region around an axis.Work Done by a Variable Force: In physics, integration is used to calculate the work done by a variable force over a distance.

Integration Rules and Formulas

The rules and formulas are pivotal, providing efficient methods for finding integrals. Here's a list that will be useful as you work through integral problems.

Power Rule: ∫ xn dx = (x^(n+1))/(n+1) + C, for n -1.
Constant Multiple Rule: ∫ k f(x) dx = k ∫ f(x) dx, where k is a constant.
Sum/Difference Rule: ∫ [f(x) g(x)] dx = ∫ f(x) dx ∫ g(x) dx.
Trigonometric Functions: Use the formulas for sine, cosine, tangent, etc., which are listed above.
Exponential Functions: ∫ e^x dx = e^x + C.
Logarithmic Functions: ∫ (1/x) dx = ln|x| + C.

Remembering vs. Deriving Integrals

While memorization has its place, it's also crucial to understand how these formulas are derived. This allows you to adapt and solve a wider range of problems.

Common Pitfalls

Here are some common mistakes to watch out for:

Forgetting the constant of integration (+ C) in indefinite integrals.
Making errors when applying the substitution method (not substituting correctly or forgetting to change the limits in definite integrals).
Misapplying the integration by parts formula (choosing u and dv incorrectly).
Errors in algebraic manipulation while simplifying the integrand.

Resources for Mastery

To become proficient, consider:

Working through a textbook and completing all practice problems.
Using online resources and practice tools (like Khan Academy and many others).
Creating your own flashcards with key formulas and examples.
Seeking help from a teacher, tutor, or study group when you encounter difficulties.

Further Exploration

After mastering these basics, consider these topics:

Improper Integrals: Integrals with infinite limits or discontinuous integrands.
Multivariable Calculus: Integration over multiple variables, useful for higher-dimensional problems.
Differential Equations: Solving equations that relate a function to its derivatives.

Mastering integration is a journey, not a destination. With persistent effort, a systematic approach, and a willingness to embrace the challenges, you can unlock the power of this vital tool. This guide offers a starting point, but the real learning happens through practice, exploration, and a curiosity to understand the underlying principles of calculus. Embrace the challenge, and the rewards will be immense.